Practice Page

Directions: Read carefully.

1.
Regarding the graphs at the right:
a) Which graph is f (x) = x2 ?
Choose:
A
B
C

b) Which graph is f (x) = 3x2 ?
Choose:
A
B
C

c) Which graph is f (x) = 0.3x2 ?
Choose:
A
B
C
PP1

 

 

2.
Regarding the graphs at the right:
a) Which graph is f (x) = x3 ?
Choose:
A
B
C

b) Which graph is f (x) = (x - 3)3 ?
Choose:
A
B
C

c) Which graph is f (x) = (x + 3)3 ?
Choose:
A
B
C
pP2

 

 

3.
The parabola f (x) = x2 - 4 is shown at the right. The minimum value of g (x) = f (x) + 3 will be:

Choose:
(0,-4)
(0,-3)
(0,-1)
pP2

 

 

4.
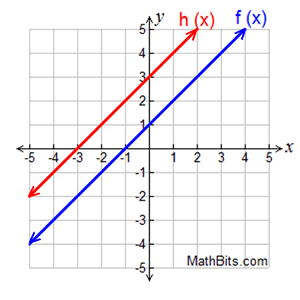
Regarding the graphs at the right:
The function h (x) can be expressed as:

Choose:
h(x) = f (x) + 2
h(x) = f (x) + 3
h(x) = 2f (x)
h(x) = 3f (x)
pp4

 

 

5.
Regarding the graphs at the right:
Which of the following statements is true?
 Choose:
g(x) is increasing on the interval
(-∞, -2)
f (x) is positive on the interval
(-∞, -2)
f (x) is decreasing on the interval
(-2, 2)
g(x) is negative on the interval
(-2, 2)
pp5

 

 

6.
Regarding the graphs at the right:
The rate of change on function g(x) is the same as the rate of change of function f (x) on the interval
1 < x < 2.

Choose:
True
False
PP6
 

 

 

7.
Regarding the graph at the right:
Which of the following statements is true?
 Choose:
The function is decreasing on the interval (0,1).
The function has an absolute maximum at its vertex point.
The function is negative on the interval (1, ∞).
As x → ∞, f (x) → ∞.
pp7

 

 

8.
Regarding the graph at the right:
a) On which interval(s) is this function increasing?
 Choose:
(-∞, -2) only
(-∞, -2) U (-3,∞)
(-2,0)
(-∞, -2) U (0,∞)

b) Does this function have relative maxima and/or relative minima?
Choose:
relative max (0,-3) and min (-2,1)
relative max +∞ and min -∞
relative max (-2,1) and min (0,-3)
no relative max or min
pp8


divider

NOTE: The re-posting of materials (in part or whole) from this site to the Internet is copyright violation
 and is not considered "fair use" for educators. Please read the "Terms of Use".