|
Up to this point, we have only flipped the functions, or shifted the positions of the functions.
Those transformations never changed the shape of the function graph,
and were referred to as
"rigid" transformations.
Now, let's try "distorting" the shapes of the functions.
These transformations will change the shape of the function graphs.
These "distorted" transformations are called "nonrigid transformations".
| Dilation: Vertical Stretch or Compress: k • f (x) |
 When a function is multiplied by a positive constant, k,
a vertical stretch, or compression, of the function will occur.
If the constant is greater than one (k > 1), a vertical stretch will occur.
If the constant is between 0 and 1, (0 < k < 1), a vertical compression will occur.
A vertical stretch, or compress, will multiply all y-values by k.
The x-values will not change.
(x, y) → (x, k • y )
 NOTE: During a vertical stretch, or compress, the root values of the function
(where y = 0), never change. They stay attached to the x-axis.
|
A vertical stretch, or compression,
transforms the "outside" (y-output values) of the function.
Vertical Stretch:
k • f (x)
where k > 1 |
A vertical shift "pulls" the graph vertically
away from the x-axis (up or down).
(It's like "pulling taffy".)
When k > 1, the graph is vertically
stretched by a factor of k.
This will multiply each of its y-coordinates by k.
Remember: The root values (where y = 0)
stay attached to the x-axis. They never change.
When working with quadratic functions, a vertical stretch makes the parabola look thinner
(the width across the parabola gets narrower).
|
 \
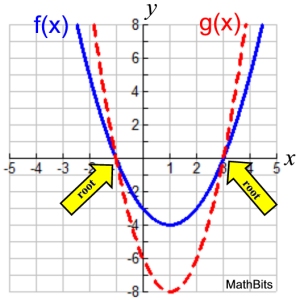
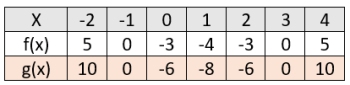
Given: f (x) = x2 and k = 2
The outputs from this stretch will be twice the
outputs from f (x). This is a scale factor of 2.
2 f (x) = 2(x2)
The new function can be renamed:
g (x) = 2 ( x2 )
|
In the example above, the x-inputs of the graphs were not changed.
During the transformation, the y-outputs of f (x) were multiplied by a factor of 2, (k = 2),
which stretched the graph f (x) vertically, creating a narrower parabola.
A vertical stretch will multiply ONLY the y-values.
Vertical Compression:
k f (x)
where 0 < k < 1 |
The difference between a vertical stretch and a vertical compress is the size of the number that is multiplied times the y-value.
If you are multiplying by a number
between zero and one, 0 < k < 1,
you are multiplying by a small fraction.
When 0 < k < 1, the graph is
vertically compressed by a factor of k.
(The graph is being squashed toward the x-axis.)
Remember: The root values (where y = 0)
stay attached to the x-axis. They never change.
When working with quadratic functions, a vertical compression makes the parabola look wider
(the parabola will look like its is flattening out
toward the x-axis.). |
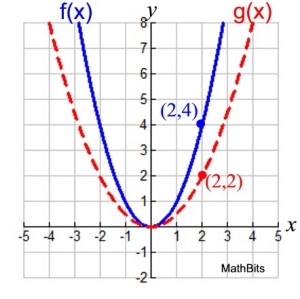
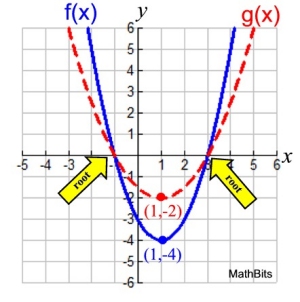
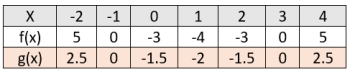
f (x) = x2 and k = ½
The outputs from this stretch will be half the
outputs from f (x). This is a scale factor of ½.
½ f (x) = ½ (x2)
The new function can be renamed:
g (x) = ½ ( x2 )
Root value (0,0) has no change.
|
In the example above, the inputs of the graphs were not changed.
During the transformation, the outputs of f (x) were multiplied by a factor of ½, (k = ½),
which compressed the graph f (x) vertically.
A vertical compression will multiply ONLY the y-values.
S U M M A R Y
| Dilations of Functions: Vertical Stretch / Compress: k • f (x) |
Vertical Stretch or Compression (Shrink)
k f (x) stretches/shrinks f (x) vertically |
"Multiply y-coordinates":
(x, y) becomes (x, ky)
"vertical dilation"
|
A vertical stretching is the stretching of the graph away from the x-axis
A vertical compression (or shrinking) is the squashing of the graph toward the x-axis.
• if k > 1, the graph of y = k•f (x) is the graph of f (x) vertically stretched by multiplying each of its
y-coordinates by k.
• if 0 < k < 1 (a fraction), the graph is f (x) vertically shrunk (or compressed) by multiplying each of its
y-coordinates by k
.
• if k should be negative, the vertical stretch or shrink is followed by a reflection across the x-axis.
|
Notice that the "roots" on the graph stay in their same positions on the x-axis. The graph gets "taffy pulled" or "pushed"
up and down from the locking root positions.
The y-values change.
|
|
|
NOTE: The re-posting of materials (in part or whole) from this site to the Internet
is copyright violation
and is not considered "fair use" for educators. Please read the "Terms of Use". |
|
