|
|
Circumference is the distance around the outside of a circle. |
|
Circumference
 |
|
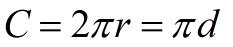Use when you know
the radius, r. |
|
Use when you know
the diameter, d. |
|
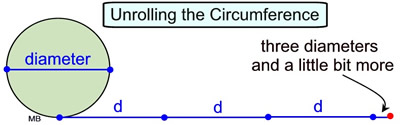
If you "unroll" the outer edge of a circle, it will form a straight segment whose length is three diameters plus a little bit more. You will get this same result using a circle with extremely small radius length as you will using a circle with enormously large radius length. It was the need to understand this constant length relationship that led to the constant pi (π = 3.14159...).
Facts about working with π (pi):
• Since C = πd, then for all circles:  .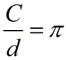
• There is no "exact" numerical value of π.
There are only numerical approximations.
π is a non-ending decimal value.
By calculator, π = 3.141592654...
Use of the full calculator value will yield the most accurate numerical answers when working with π.
|
|
When working with a formula containing π, there are two ways to express the answer.
• The EXACT answer: where π appears in the answer, such as Answer = 6π.
• The APPROXIMATE answer: where a calculator is used to approximate the value of π, such as Answer = 18.84955592. |
When problems are dealing with π, an indication will usually be given as to whether express the answer as an "exact" answer or as an "approximation". Approximations usually indicate that the answer should be rounded to the nearest integer, or rounded to the nearest tenth, etc. A problem may state to use 3.14 as an approximation of π.
CAUTION: 3.14 is only an approximation of π. It does not equal π .
CAUTION: The fraction 22 over 7 is not equal to pi.
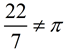
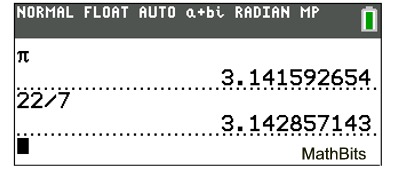
If no directions for expressing the answer are given,
state the exact answer,
or state the answer using the full calculator value.
 Find the circumference of a circle with a radius of 14 inches.
Express answer rounded to the nearest hundredth.
|
Since the radius is given, use the formula:
C = 2πr
C =
2π(14) = 28π = 87.9645943 = 87.96
Circumference = 87.96 inches |
While decorating the gym for the spring dance, students decided to place a string of daisies around the clock on the gym wall. If the string of daisies needs to be 38 inches, what is the diameter of the clock to the nearest tenth of an inch?
|
Since the diameter is needed, use the formula:
C = πd
38 =
πd
d = 12.09477567=12.1
diameter = 12.1 inches |
A bicycle tire has a radius of 18 inches. How far will the tire turn in one revolution?
|
The length of one revolution will be the length of the circumference.
Since the radius is given, use the formula:
C = 2πr
C =
2π(18) = 36π inches
or 113.0973355 inches |
NOTE: The re-posting of materials (in part or whole) from this site to the Internet
is copyright violation
and is not considered "fair use" for educators. Please read the "Terms of Use". |
|
|
