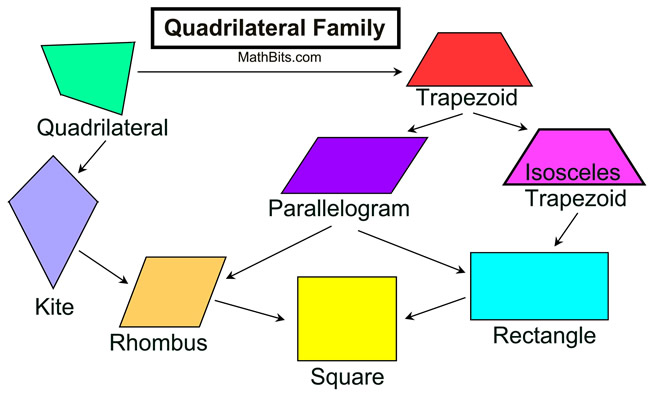
Quadrilateral
A quadrilateral is ANY four-sided figure.
|
Do not assume any additional properties unless you are given additional information.
Properties:
• has exactly four sides
• the sum of the interior angles is always 360º
|
Kite

A kite is a quad with
2 sets of adjacent, congruent sides.
|
In a kite, the congruent sides are adjacent (or consecutive) and not opposite one another. (Kites referred to on this site are convex kites.) Properties:
• has two distinct pairs of adjacent sides congruent
• the diagonals are perpendicular
• has one pair of opposite angles congruent
• one diagonal forms two isosceles triangles
• one diagonal forms two congruent triangles
• one diagonal bisects the angles
• one diagonal bisects the other diagonal
|
Trapezoid
A trapezoid is a quadrilateral with at least one pair of parallel sides.
|
This site uses the definition that a trapezoid has "at least" one pair of parallel sides. This is called the "inclusive" definition.
Properties:
• has a least one pair of parallel sides
• the median of a trapezoid is parallel to the bases and ½ the sum of the lengths of the bases
|
Isosceles
Trapezoid

An isosceles trapezoid is a trapezoid with congruent base angles.
|
Be sure that you are given (or can prove) that a trapezoid is isosceles. Never "assume" it is isosceles by looking at the diagram.
Properties:
• a trapezoid with one set of parallel sides
• has congruent legs
• has base angles congruent
• has congruent diagonals
• has opposite angles supplementary
|
Parallelogram

A parallelogram is a quadrilateral with both pairs of opposite sides parallel. |
Notice that these properties of a parallelogram come in sets of twos: 2 about sides, 2 about angles, and 2 about diagonals.
Properties:
• has two pair of opposite sides parallel
• has two pair of opposite sides congruent
• has opposite angles congruent
• had consecutive angles supplementary
• diagonals bisect each other
• diagonals form two congruent triangles
|
Rectangle

A rectangle is a parallelogram with 4 right angles.
|
Notice that you only need to add two additional properties to a parallelogram
to describe a rectangle.
Properties:
A rectangle has all of the properties of a parallelogram PLUS:
• has four right angles
• has congruent diagonals
|
Rhombus
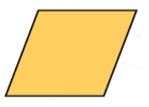
A rhombus is a parallelogram with all 4 sides congruent.
|
Notice that adding 3 additional properties to a parallelogram will describe a rhombus. A rhombus is a slanted square.
Properties:
A rhombus has all of the properties of a parallelogram PLUS:
• has four congruent sides
• has diagonals that bisect the angles
• has perpendicular diagonals |
Square

A square is a parallelogram with 4 congruent sides and 4 right angles. |
The square is the most specific member of the quadrilateral family. It has the largest number of properties.
Properties:
A square has
•
all of the properties of a parallelogram PLUS
•
all of the properties of a rectangle PLUS
•
all of the properties of a rhombus |