|
The Multiplication Rule of Probability is used to find
the probability that event A and event B both occur.
The final solution will depend upon whether the two events are independent events, where one event does not affect the other. |
|
In probability, an outcome is in event "A and B"
only when the outcome is in both event A and event B.
(Intersection)
|
|
In a Venn Diagram, an element is in the intersection of "A and B" only when the element is in BOTH sets. |
"AND" is associated with the concept of "BOTH", intersection (overlapping).
You are looking for elements in the sample spaces for events A and B that overlap.
The notation  is used for AND (intersection). is used for AND (intersection).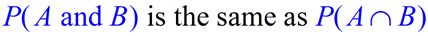
Rules (for AND):
(may be referred to as the "Probability Multiplication Rule"
and is applied when more than one event occurs in succession.)

(when A and B are independent events)
P(A and B) = P(A) • P(B | A)
(when A and B are dependent - if A occurs first,
P(A and B) = P(A) • P(B, once A has occurred)
P(A and B) = P(B) • P(A | B)
(when A and B are dependent - if B occurs first,
P(A and B) = P(B) • P(A, once B has occurred)
|
|
 The rule for "AND" must take into account whether the events affect one another. The rule for "AND" must take into account whether the events affect one another.
Actually, there is really only ONE rule, the second rule.
If sets A and B are independent, P(B | A) = P(B) or P(A | B) = P(A).
So, P(A and B) = P(A) • P(B | A) = P(A) • P(B) when A and B are independent.
Or, P(A and B) = P(B) • P(A |B) = P(B) • P(A) when A and B are independent.
 |
. |
A die is rolled and a penny is tossed. What is the probability that a 3 is rolled on the die and a head is tossed on the penny? |
Solution:
Sample space (die) = {1, 2, 3, 4, 5, 6}
Event A: Rolling a 3 on a die: P(A) = 1/6
Sample space (penny)= {H, T}
Event B: Tossing a head with the penny: P(B) = 1/2
Probability of BOTH: These are independent events. Event A does not have an effect on event B.
Answer: P(A and B) = P(A) • P(B) = 1/6 • 1/2 = 1/12 |
 |
 |
. |
Two cards are drawn from a standard deck of cards. What is the probability that the first card is a club and the second card is a heart?
The first card was not put back into the deck after being drawn. |
Solution:
Sample space = {52 cards in the deck}
Event A: Drawing a club: {13 clubs} P(A) = 13/52 = 1/4
Sample space = {51 cards - one card in now missing}
Event B: Drawing a heart: {13 hearts} P(B) = 13/51
Probability of BOTH: These are dependent events. Event B is affected by event A. Without replacing the card, the sample space for the second draw is changed to only 51 available cards.
Answer: P(A and B) = P(A) • P(B | A) = 13/52 • 13/51 = 169/2652
|
 Dependent events since the first card was not replaced.
Dependent events since the first card was not replaced. |
 |
. |
A sack contains 4 green marbles, 3 blue marbles and 2 red marbles. What is the probability of drawing two green marbles in a row if the first marble is returned to the sack before the second draw (with replacement)? |
Solution:
Sample space = {9 marbles}
Event A: Drawing a green marble: P(A) = 4/9
Sample space = {9 marbles}
Event B: Drawing a green marble: P(B) = 4/9
Probability of BOTH: These are independent events. Event B is not affected by event A since the first marble is replaced.
Answer: P(A and B) = P(A) • P(B) = 4/9 • 4/9 = 16/81
|
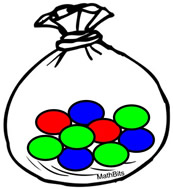 Independent events since the first marble was replaced.
Independent events since the first marble was replaced. |

NOTE: The re-posting of materials (in part or whole) from this site to the Internet
is copyright violation
and is not considered "fair use" for educators. Please read the "Terms of Use". |
|
|