|
Multiplying and dividing radicals makes use of the "Product Rule" and the "Quotient Rule" as seen at the right. The "n" simply means that the index could be any value. The examples on this page use square and cube roots.
 Multiplying Radicals
Multiplying Radicals |
When multiplying radicals (with the same index), multiply under the radicals, and then multiply any values directly in front of the radicals. |
|
|
|
ANSWER: 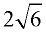 |
| |
Multiply the values under the radicals. 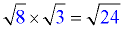
Then simplify the result. 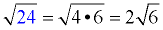
|
|
|
ANSWER: 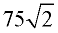
|
| |
Multiply out front and multiply under the radicals. 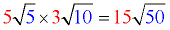
Then simplify the result.
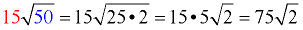
|
|
Product Rule
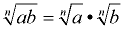 where a ≥ 0, b≥ 0 where a ≥ 0, b≥ 0
"The radical of a product is equal to the product of the radicals of each factor." |
Quotient Rule
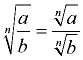
where a ≥ 0, b > 0
"The radical of a quotient is equal to the quotient of the radicals of the numerator and denominator."
|
|
 Dividing Radicals
Dividing Radicals |
When dividing radicals (with the same index), divide under the radical, and then divide the values directly in front of the radical. |
|
|
|
|
ANSWER: 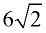 |
| |
Divide out front and divide under the radicals. 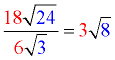
Then simplify the result. 
|
|
|
ANSWER: 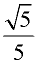 |
| |
This fraction will be in simplified form when the radical is removed from the denominator. If we create a perfect square under the square root radical in the denominator the radical can be removed. To do so, we multiply the top and bottom of the fraction by the same value (this is actually multiplying by "1"). If we multiply by the square root radical we are trying to remove (in this case multiply by  ), we will have removed the radical from the denominator. ), we will have removed the radical from the denominator.
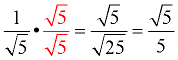 You have just "rationalized" the denominator! You have just "rationalized" the denominator!
You turned an irrational value into a rational value in the denominator.
|
 |
 |
ANSWER: 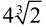 |
| |
We need to "rationalize the denominator". When the denominator is a cube root, you have to work harder to get it out of the bottom. Unfortunately, it is not as easy as choosing to multiply top and bottom by the radical, as we did in Example 2. Here is why:
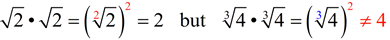
In the first case, the power of 2 and the index of 2 allow for a perfect square under a square root and the radical can be removed.
In the second case, the power of 2 with an index of 3 does not create an inverse situation and the radical is not removed. We need an additional factor of the cube root of 4 to create a power of 3 for the index of 3.
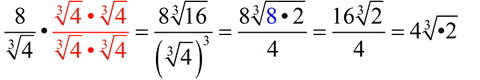
It may be the case that the radicand of the cube root is simple enough to allow you to "see" two parts of a perfect cube hiding inside. In this case, you can simplify your work and multiply by only one additional cube root. As shown below, one additional factor of the cube root of 2, creates a perfect cube in the radicand.
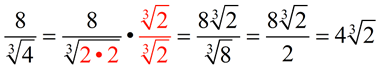
|
 |
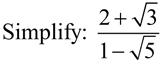 |
ANSWER: 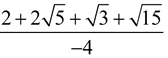 |
| |
We will use a conjugate to rationalize the denominator! The problem with this fraction is that the denominator contains a radical. As such, the fraction is not considered to be in simplest form. As we saw in Example 8 above, multiplying a binomial times its conjugate will rationalize the product. This process will remove the radical from the denominator in this problem ( if we multiply the denominator by 1 + 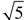 ). So as not to "change" the value of the fraction, we will multiply both the top and the bottom by 1 + ). So as not to "change" the value of the fraction, we will multiply both the top and the bottom by 1 + 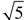 , thus multiplying by 1. , thus multiplying by 1.

While the numerator "looks" worse, the denominator is now a rational number and the fraction is deemed in simplest form. Notice that there is nothing further we can do to simplify the numerator.
Did you notice how the process of "rationalizing the denominator" by using a conjugate resembles the "difference of squares": a2 - b2 = (a + b)(a - b)? They both create perfect squares, and eliminate any "middle" terms.
|
 |
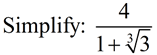 |
ANSWER: 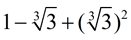 |
|
While the conjugate proved useful in the last problem when dealing with a square root in the denominator, it is not going to be helpful with a cube root in the denominator. Watch what happens when we multiply by a conjugate:
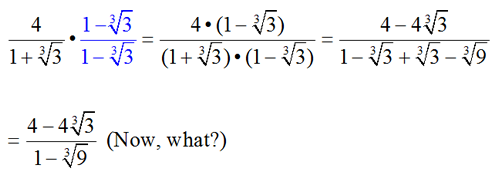
The cube root of 9 is not a perfect cube and cannot be removed from the denominator. Instead of removing the cube root from the denominator, the conjugate simply created a new cube root in the denominator.
To solve this problem, we need to think about the "sum of cubes formula":
a3 + b3 = (a + b)(a2 - ab + b2). This formula shows us that to obtain perfect cubes we need to multiply by more than just a conjugate term.
Let a = 1 and b = the cube root of 3. We will multiply top and bottom by
a2 - ab + b2.
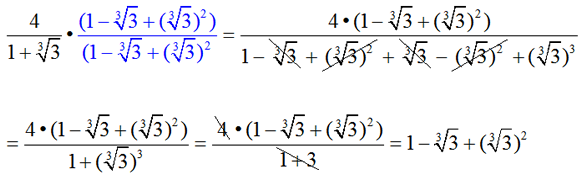
WOW!
Note: If the denominator had been 1 "minus" the cube root of 3, the "difference of cubes formula" would have been used: a3 - b3 = (a - b)(a2 + ab + b2).
|

| Expressions with Variables |
 |
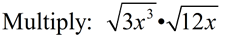 |
ANSWER: 6x2 |
| |
Multiplying will yield two perfect squares.

|
 |
 |
ANSWER: 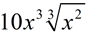 |
| |
Search out the perfect cubes and reduce. This will simplify the multiplication. If you do not "see" the perfect cubes, multiply through and then reduce.
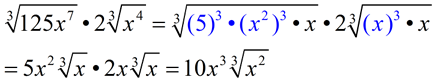
|
 |
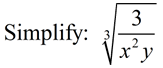 |
ANSWER: 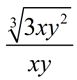 |
| |
Using the approach we saw in Example 3 under Division, we multiply by two additional factors of the denominator. Look for perfect cubes in the radicand as you multiply to get the final result.
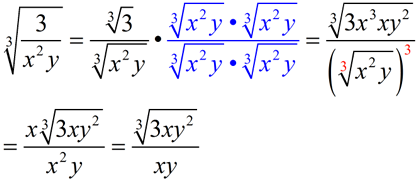
Or, another approach is to create the simplest perfect cube under the radical in the denominator.
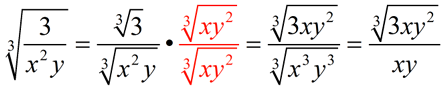
|
|
|