Practice Page
Directions: Read carefully and choose the best answer.

1.
Find the focus and directrix of the parabola y = ¼ x2.
paraguy1
Choose:
 
focus: (0,2); directrix: y = -2
  focus: (0,¼); directrix: y = -¼
  focus: (0,1); directrix: y = -1
  focus: (0,4); directrix: y = -4



2.
Write the equation of a parabola with a focus of (0,-2) and a directrix y = 2.
paraguy4
Choose:
 
y = oneover8x2
 
y2 = -4x
  y = 1over8pox2
  y2 = -8x



3.
Find the focus and directrix of the parabola y - 3 = -(x + 2)2.
paraguy2
Choose:
 
focus: (-2,3); directrix: y = -3
 
focus: (-2,3¼); directrix: y = 2¾
  focus: (-2,2¾); directrix: y = 3¼
  focus: (-2,3); directrix: y = 4



4.
Write the equation of a parabola with a vertex of (-1,4) and a directrix of x = 1.
paraguy3
Choose:
 
(x + 1)2 = 2(y - 4)
 
(x - 1)2 = -2(y + 4)
  (y + 4)2 = 8(x - 1)
  (y - 4)2 = -8(x + 1)



5.
Given parabola x = (y + 5)2 + 2. What is the equation of its directrix?
paraguy5
Choose:
 
x = 2
 
x = 1¾
  y = -5
  y = 5¼



6.
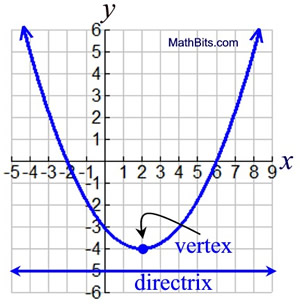
Given the graph shown at the right, find the equation of the parabola. (You may assume that the vertex coordinates and the directrix equation have integer values.)
Choose:
 
(x + 2)2 = 4(y - 4)
 
(x + 2)2 = (y - 4)
 
(x - 2)2 = 4(y + 4)
 
(x - 2)2 = (y + 4)

parabolablue



7.
This NASA tracking antenna is a parabolic dish. Its receiver is placed at its focus 27 meters from the center of the dish (its vertex). For this problem, assume the dish is pointing upward and represent the vertex as (0,0). Write a parabolic equation that models the cross section of the antenna dish.
Choose:
 
x2 = 27y
 
x2 = 54y
 
x2 = 108y
 
y2= 54x
DSN antennaPhoto courtesy of NASA.
This is a 70 meter tracking antenna (with a parabolic dish) weighing nearly 2,970 ton.



8.
A parabola has its vertex at the origin and focus at (0,4). Find the length of the segment that passes through the focus, is parallel to the directrix, and whose endpoints lie on the parabola.
paraguy6
Choose:
 
length = 8 units
 
length = 16 units
  length = 32 inches
  length = 64 units



9.
Write the equation of a parabola with a focus of (-1,-2) and a directrix y = -4.
paraguy7
Choose:
 
(x + 1)2 = 4(y + 3)
 
(x+ 1)2 = ¼(y + 3)
  (x - 1)2 = 4( y - 3)
  (x - 1)2 = ¼(y - 3)



10.
Which of the following parabolic equations could be used to represent the graph at the right?
(Check all that apply, and hit SUBMIT!)
 

x2 - 8x - 2y + 18 = 0

(y - 1)2 = 2(x - 4)

y = ½(x - 4)2 + 1

x2 - 8x - 2y + 14 = 0

y = ½x2

2(y - 1) = (x - 4)2

(y - 1)2 = ½(x - 4)

        

parabolaex10blue

 

divider

NOTE: The re-posting of materials (in part or whole) from this site to the Internet is copyright violation
 and is not considered "fair use" for educators. Please read the "Terms of Use".