The word "commute" is defined as "to travel back and forth on a regular basis".
Example: Karl commutes to the local community college campus.

Think of the elements as "commuting" from one location to another.  "They get in their cars and drive to their new locations."  This explanation will help you to remember that the elements are "moving" (physically changing places).

The word "associate" is defined as "to join as a partner, friend, or companion"
Example: Tina and Karly were closely associated with each other during college.

The associative property can be thought of as illustrating "friendships" (associations).  The parentheses show the grouping of two friends.  In the example below, the girl (Y) decides to change from the green-shirted boyfriend (X) to the blue-shirted boyfriend (Z).   "I don't want to associate with you any longer!"  Notice that the elements do not physically move, they simply change the person with whom they are "holding hands" (illustrated by the parentheses.) 

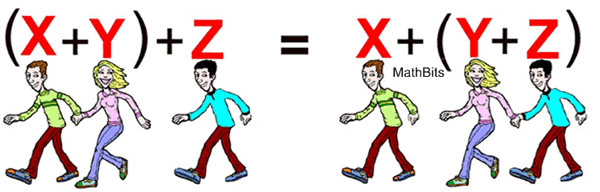
The word "distribute" is defined as "to deal out or occur throughout".
Example: Campaign buttons are being distributed to students and teachers.

Let's consider the problem 3(x + 6).  The number in front of the parentheses is "looking" to distribute (multiply) its value with all of the terms inside the parentheses. 

The word "identity" is defined as "the state or fact of remaining the same".
Example: The identity of the fingerprints at the crime scene helped catch the burglar.

Try to remember the "I" in the word identity.  Certain variables can have an "attitude" such as, "I am the most important thing in the world and I do not want to change!"  The identity element allows the variable to maintain this attitude and remain the same.

The word "inverse" is defined as "something that is the opposite or reverse".
Example: His idea is the inverse of what is commonly thought to be true.

Think of the inverse as "inventing" an identity element. What would you need to add (or multiply) to the given element to turn it into an identity element?