1. Start with a quadratic equation set = 0.

2. Move the constant to the right side.

3. Divide each term by a to get the leading coefficient to be one.

4. Get ready for a perfect square trinomial on the left side (put in the boxes).

5. To find the needed value, take half of the coefficient of the x-term, 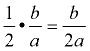, and square it, 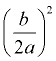. Add this value to both sides of the equation (put it in the boxes).

6. Square the last term on the right side. Find a common denominator on the right.

7. Combine terms on the right, placing b² in front of -4ac in the numerator.

8. Re-write the perfect square trinomial on the left side as the square of a binomial.

9. Take the square root of both sides. Simplify the radical on the right side.

10. Subtract the constant away from x. Write as a single fraction on the right side.