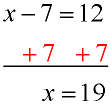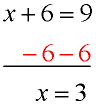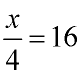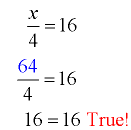Solving One-step Linear Equations MathBitsNotebook.com Topical Outline | Algebra 1 Outline | MathBits' Teacher Resources Terms of Use   Contact Person: Donna Roberts

Linear equations are equations of degree one. (The variable has an exponent of one.)

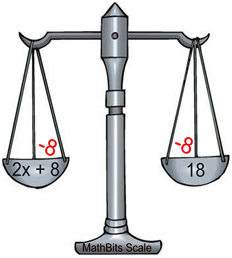
Solving a linear equation is a process of undoing operations that have been applied to the variable to create the equation. Your goal is to isolate the variable on one side of the equal sign. Linear equation: 2x + 8 = 18 Remember that the "equal sign" implies that the expressions on either side are equivalent. You can think of both expressions as "weighing" the same amount. You must be careful, when solving for the variable, to maintain an equal "weight" on both sides. This process of "balancing the equation" is accomplished by always making the same changes to BOTH sides of the equal sign.

Solving one-step equations: (undo operation by either add or subtract) Solve for x:    x - 7 = 12 Analysis: We need to isolate the variable x. Since the number 7 has been subtracted from x, we will need to do the opposite and add 7, to get rid of the -7. This process is actually using the additive inverse property to create a 0 on the left side (-7 + 7 = 0), and then using the additive identity to isolate the x, since x - 0 = x. Remember to add the 7 to BOTH sides of the equation, to keep the equation balanced. Check answer: A nice bonus to solving equations is that you always know if you have the correct answer. Simply substitute your answer into the original equation and see if the result is true. Check: x - 7 = 12 19 - 7 = 12 12 = 12 True!   Solve for x:    x + 6 = 9 Analysis: We need to isolate the variable x. Since the number 6 has been added to x, we will need to do the opposite and subtract 6, to get rid of the 6. This process is actually using the additive inverse property to create a 0 on the left side (6 - 6 = 0), and then using the additive identity to isolate the x, since x + 0 = x. Remember to subtract 6 from BOTH sides of the equation, to keep the equation balanced. Check answer: A nice bonus to solving equations is that you always know if you have the correct answer. Simply substitute your answer into the original equation and see if the result is true. Check: x + 6 = 9 3 + 6 = 9 9 = 9 True!   Solving one-step equations: (undo operation by multiply, or divide by non-zero) Solve for x: 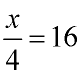   Analysis: We need to isolate the variable x. Since x has been divided by 4, we will need to do the opposite and multiply by 4, to get rid of the 4. This process is actually using the multiplicative inverse property to create a 1, and then using the multiplicative identity to isolate the x, since 1•x = x. This problem could also be solved by dividing both sides by 1/4 (which is the same as multiplying by 4). But, why make the problem more difficult! Remember to multiply BOTH sides of the equation by 4, to keep the equation balanced. Check answer: A nice bonus to solving equations is that you always know if you have the correct answer. Simply substitute your answer into the original equation and see if the result is true. 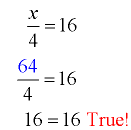Check:   Solve for x:    5x = 125 Analysis: We need to isolate the variable x. Since the number 5 has been multiplied times x, we will need to do the opposite and divide by 5, to get rid of the 5. This process is actually using the multiplicative inverse property to create a 1, and then using the multiplicative identity to isolate the x, since 1•x = x. This problem can also be solved by multiplying both sides by 1/5 (which is the same as dividing by 5). Remember to divide BOTH sides of the equation by 5, to keep the equation balanced. Check answer: A nice bonus to solving equations is that you always know if you have the correct answer. Simply substitute your answer into the original equation and see if the result is true. Check: 5x = 125 5(25) = 125 125 = 125 True!

In summary, we have seen that solving equations is the process of "undoing" what has been done to the variable. This process is known as solving equations by "inverse" operations. Addition and subtraction are "inverse" operations (by application of the additive inverse property).
Multiplication and division are "inverse" operations (by application of the multiplicative inverse property). These properties are used to isolate the variable, thus solving the equation.

For calculator help with Solving Equations click here.