Pattern Rule: To find the factors, we need two values that will multiply to the constant term and add to the coefficient of the middle term.
If this trinomial is factorable, we know that there must be two factors of 7 that will add to +2 (since all signs are positive).
Factors of 7:
7 is prime, so we only have 7 • 1
The sum of 7 and 1 is NOT 2.
This trinomial can not be factored.
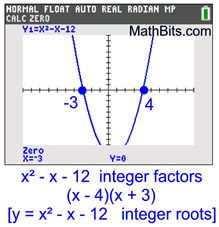
Examine the graph of y = x2 + 2x + 7.

This graph does not even intersect with the x-axis.
The expression is not factorable over the set of integers.
|
Factoring by Grouping: To find the factors to split the middle, we need values that multiply to a • c but add to b.
a • c = 3 • 5 = 15
b = -1
Factors of 15:
(one must be positive and one negative)
Sum of possible factors of 15:
+ 15 + -1 = +14
-15 + +1 = -14
5 + -3 = 2
-5 + 3 = -2
No choice adds to -1.
This trinomial can not be factored.
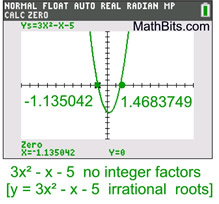
While this graph crosses the x-axis, it crosses at irrational values, whose factors will not be factorable over the set of integers.
The expression is not factorable over the set of integers.
|