|
Directions: Answer these questions pertaining to the graphs of polynomial functions. Choose the best answer. The cartoon "comments" may, or may not, be helpful!
1. |
Given: P(x) = x2 + 2x - 24
At what x-values will the graph cross the x-axis? |
|
|
|
2. |
A polynomial function is defined to be
f (x) = (x + 3)(x - 2)2.
Which choice describes the graph's behavior at the x-axis? |
|
|
|
|
|
3. |
Given: the graph at the right.
Which choice lists possible "factors" of this polynomial function?
|
|
|
|
4. |
Given: the graph at the right.
Which polynomial function may represent this graph?
Choose:
|
|
|
|
5. |
Given: the graph at the right.
Which choice lists possible "roots" of this polynomial function?
Choose:
|
|
|
6. |
Given: P( x) = x3 + 6 x2 + 8 x
Which choice shows the roots of this polynomial function? |
| |
|
|
|
7. |
Given: P(x) = x2 - 2x - 48
Which choice shows the x-intercepts of this polynomial function?
|
 |
| |
|
8. |
Given: P(x) = x2 - 6x + 9
Which choice states the number of x-intercepts of this polynomial function?
|
|
|
|
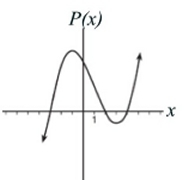
9.
|
Based upon the sketch at the right, which expression may have been used to create P(x)?
|
 |
|
|
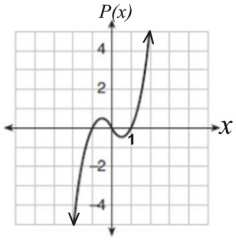
10.
|
By examining the roots of the graph at the right, write a possible equation, P(x), that could produce this graph. |
 |
|
|

NOTE: The re-posting of materials (in part or whole) from this site to the Internet
is copyright violation
and is not considered "fair use" for educators. Please read the "Terms of Use". |
|
|