|
For any non-zero number x, and for any positive integer n,
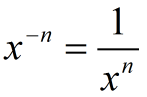 |
An expression raised to a negative exponent is equal to one divided by the expression with the sign of the exponent changed to positive.
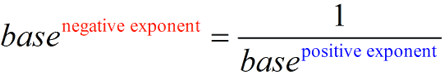
|

|
Remember, any number (or expression) with a negative exponent ends up
on the opposite side of the fraction bar as a positive exponent.
 Generally, we do not leave exponential expressions with negative exponents.
Generally, we do not leave exponential expressions with negative exponents.
When asked to simplify an exponential expression, write the answer without negative exponents.
The Product Rule for Exponents states that xm • xn = xm+n.
"When multiplying exponential expressions, if the bases are the same, add the exponents."
If we apply this law to work with a negative exponent, we get 43 • 4-3 = 43+(-3) = 40 = 1.
This application shows us that
43 • 4-3 = 1, which means that 4-3 must the multiplicative identity of 43. Therefore, 4-3 must be a fraction and it must be the reciprocal of 43.
Consequently, 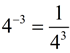 . .

See how the Quotient Rule for Exponents supports the Negative Integer Exponent Rule:
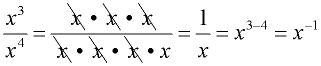

There are three important concepts at work in this Rule: |
For any non-zero number x, and for any positive integer, n:

The use of a positive exponent is an application of repeated multiplication by the base:
43 = 4 • 4 • 4 = 64.
The use of a negative exponent produces the opposite of repeated multiplication.
It can be thought of as a form of repeated division by the base:
4-3 = 1 ÷ 4 ÷ 4 ÷ 4 = 0.015625
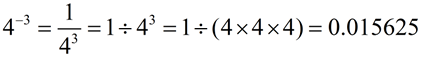
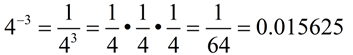

Examples: (numerical and algebraic applications)
1. 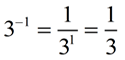
The negative 1 exponent indicates that the value is the same as 1 over 3 to a power of positive 1. |
2. 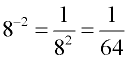
|
3. 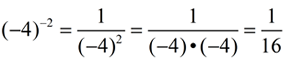
Be sure to keep the negative base in a set of parentheses to avoid calculation errors.
|
4. 
Be careful with values not being affected by the exponent (in this case the 6).
|
5. 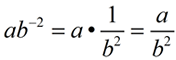
Sneaky one!!!! Remember that the exponent belongs only to the base value to which it is attached. In this problem, the exponent is not attached to the variable "a". If it was to be attached to "a", a parentheses would be used.
(ab)-2
|
6. 
Each variable has its own assigned exponent, so they are treated separately. Combing the results to form the final answer. |
7. Negative exponent in denominator.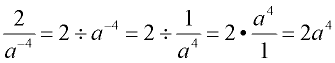
Remember that the "FRACTION BAR" means division. Apply the rule for dealing with a negative exponent. Don't forget to "invert" the second term when dividing. |
8. Working with Power to Power Rule:
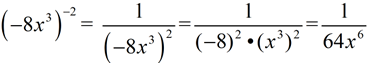
The power of -2 in this problem affects both the -8 and the x3. This example also shows the Power to Power Rule for (x3)2 = x6,
|
9. Negative exponent with Quotient Rule:

Numerical Problem: The Quotient Rule subtraction is always done "top" minus "bottom" exponents. In this problem we get 3 - 5 = -2. This gives us a negative exponent. Remember, with negative exponents, the answer becomes one over the base with the exponent changed to positive. |
10. Negative exponent with Quotient Rule: 
Algebraic Problem: Again, subtraction "top" minus "bottom" exponents. In this problem we get 5 - 9 = -4.
The answer becomes one over the base of x raised to the power of +4. |
11. 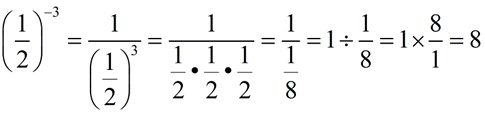
WOW!!
This problem combines a multitude of skills to arrive at the final answer. Don't forget to deal with the complex fraction of 1 over 1/8. |
HINT: When working with negative exponents, the negative exponent is telling you that the factor is on the wrong side of the fraction bar. Simply move that factor to the other side of the fraction bar, and remove the negative sign from the exponent. |
|
|
|