Proof for Theorem (converse)
Prove: If a parallelogram has perpendicular diagonals, it is a rhombus.
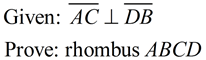thrh4
thrhombus1
 
Statements
  
Reasons
1.
thrhombus313;pf1
1.
Given
2.
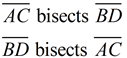thrh43
2.
Diagonals of a parallelogram bisect each other.
3.
thrhombus34
3.
A segment bisector forms two congruent segments.
4.
∠AED; ∠DEC, ∠CEB, ∠AEB right angles
4.
Perpendicular lines meet to form right angles.
5.
∠AEDcongruent∠DECcongruent∠CEB congruent∠AEB
5.
 All right angles are congruent.
6.
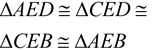thrh45
6.
SAS - if 2 sides and the included ∠ of one Δ are congruentto the corres. parts of another Δ, the Δs are congruent.
7.
thrhombus13
7.
CPCTC - corres. parts of congruentΔs are congruent.
8.
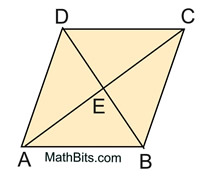
rhombus ABCD
8.
A rhombus is a parallelogram with 4 congruent sides.

All Rights Reserved - Copyright MathBitsNotebook.com