|
When you worked with systems of equations, you saw that two coplanar lines
(in the same plane) can be arranged in three different situations.
Coincide (Coincident)
Lines that coincide lie one on top of the other. They are the SAME line with the equations expressed in different forms. If two coincident lines form a system, every point on the line is a solution to the system.

|
Parallel
Lines in a plane that are parallel, do not intersect. Two lines are parallel if they have the same slope, or if they are vertical. If two parallel lines form a system, there are no solutions to the system.
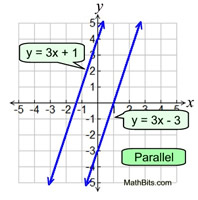
|
Intersect
If lines intersect, the lines cross in one point. The angles at which the two lines intersect can vary. An intersection point creating a 90º angle forms a perpendicular. If two intersecting lines form a system, there is one solution.
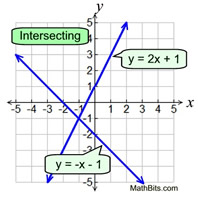
|
When we work in three dimensions, we will add another situation to our investigation of lines.
Skew lines are lines that are not coplanar, do not intersect, and are not parallel.
Skew lines exist only in three or more dimensions.

Let's start our investigation of lines by examining parallel lines.
|
Parallel lines are coplanar lines (in the same plane) that never intersect (never cross each other). |
|
The slope of a line measures its steepness (or its angle from the horizontal).
Lines that are parallel have the same steepness (or the same angle from the horizontal).
Since parallel lines have the same steepness, they have the same slope.
Non-vertical parallel lines have the same slopes!
The slopes are equal. |
Why did we specify "non-vertical" parallel lines?
In the coordinate plane, all vertical lines are parallel to the y-axis, and are parallel to one another. But, the slopes of vertical lines are undefined since vertical lines have no "run".
A "run" of zero
causes the "rise/run" fraction to have a zero denominator. When discussing numerical slopes, we cannot mathematically say that an "undefined" slope equals (or is the same as) another "undefined" slope.
Parallel lines are marked with "feathers" (similar to what you see on an archery arrow) to show that they are parallel. The "feathers" look like "greater than" symbols on the lines.
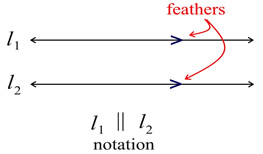
|
 |
Equations of Lines:
y = 2x + 1
y = 2x - 5
y = 2x + 1.3
y = 2x |
 |
All of the lines at the left are parallel.
They all have the same slope (m).
(y = mx + b) |

Proving Slope Criteria for Parallel Lines:
Let's prove that parallel lines have equal slopes, AND that equal slopes imply parallel lines.
We will look at a "Geometric Proof" and at an "Algebraic Proof".
|
If two distinct lines are parallel, the slopes of the lines are equal. |
|
Vertical lines will not be considered since their slopes are undefined and cannot be considered equal. If the lines are parallel horizontal lines, the slopes are both zero. Now let's consider all lines that are not vertical and not horizontal.
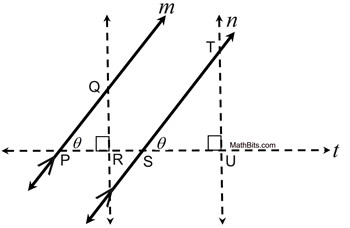
Given: Two distinct parallel lines m and n.
Prove: The slope of m = the slope of n
We will be drawing auxiliary lines and constructions to complete this proof. |
 |
|
Reasons |
1. m || n |
1. Given |
| 2. Draw a transversal line, t,
intersecting
at P and S. |
2. Two points determine one line. |
3. Construct two perpendiculars to t that
intersect m at Q and n at T. |
3. From a point not on a line, only one perpendicular may be constructed. |
4. ∠ QPR 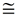 ∠ TSU |
4. If 2 lines are ||, the corresponding angles are congruent. |
5.∠PRQ, ∠SUT are right angles |
5. Perpendicular lines form rt. angles. |
6. ∠ PRQ 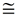 ∠ SUT |
6. All right angles are congruent. |
7. Δ PRQ Δ SUT |
7. AA for similar triangles |
8. 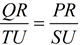 |
8. Corresponding sides of similar triangles are in proportion. |
9. 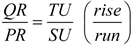 |
9. Property of proportions (alternation). |
10. slope of m = slope of n |
10. Definition of slope (rise/run). |

 |
Since we are trying to establish a connection between parallel lines and equal slopes, we will need to also prove the converse of the theorem stated above. In this manner, we will connect parallel lines to equal slopes AND equal slopes to parallel lines. |
|
If the slopes of two distinct lines are equal, the lines are parallel. |
|
If the slopes of the lines are both zero, the lines are horizontal and are parallel by definition. Since the slopes of vertical lines are undefined and not considered equal, vertical lines will not be considered.
Given: Two distinct lines m and n with equal slopes.
Prove: m || n
We will be drawing auxiliary lines and constructions to complete this proof. |
 |
|
Reasons |
1. lines m and n with equal slopes |
1. Given |
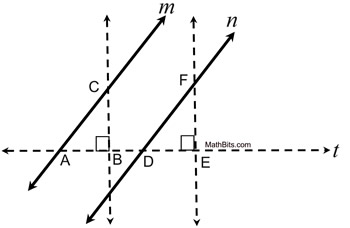
2. Draw a transversal line, t,
intersecting
at A and D. |
2. Two points determine one line. |
3. Choose point B. Copy  at D, labeling E, such that AB = DE. |
3. A segment may be copied, maintaining its length. |
4. Construct 2 perpendiculars to t at B and E. |
4. From a point on a line, only one perpendicular may be constructed. |
5. 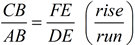 |
5. Definition of slope (rise/run). |
6. CB • DE = AB • FE |
6. In a proportion, product of means equals product of extremes. |
7. CB • AB = AB • FE |
7. Substitution |
8. CB = FE |
8. Division by AB |
9. 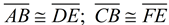 |
9. Congruent segments have = length. |
10. Δ ABC 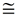 Δ DEF |
10. SAS for congruent triangles |
11. ∠ BAC 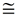 ∠ EDF |
11. Corresponding parts of congruent triangles are congruent. |
12. m || n |
12. If 2 lines are cut by a transversal and the corresponding angles are congruent, the lines are parallel. |
|

|
If two distinct lines are parallel, the slopes of the lines are equal. |
|
Vertical lines will not be considered since their undefined slopes cannot be equal.
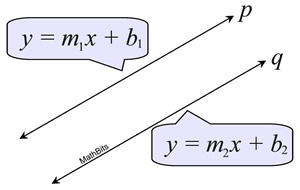
We are given that p || q.
We can write a linear equation to represent each line.
For line p, y = m1x + b1, and for line q, y = m2x + b2.
If lines are parallel, they share no common points. We saw this when we worked with systems of equations. Parallel systems had no solutions. So if we set these two equations equal, and try to solve for x, there must be no solution. Let's see what happens: |
 |
m1x + b1 = m2x + b2
m1x - m2x = b2 - b1
x(m1 - m2) = b2 - b1
The only way there can be no solution for x is for m1 - m2 to equal zero.
m1 - m2 = 0
This gives us
m1 = m2 and the slopes are equal. |

 |
As was done in the Geometric Proof, we need to also prove the converse of the theorem. In this manner, we will connect parallel lines to equal slopes AND equal slopes to parallel lines. |
|
If the slopes of two distinct lines are equal, the lines are parallel. |
|
If the slopes of the lines are both zero, the lines are horizontal and are parallel by definition. Since the slopes of vertical lines are undefined and not considered equal, vertical lines will not be considered.
We are given that m1 = m2.
We can write a linear equation to represent each line.
For line p, y = m1x + b1, and for line q, y = m2x + b2.
If p and q are distinct (different) lines, they will not coincide (be the same line). This means that b1 cannot be the same as b2 because if they are, the lines will be the exact same line. So we know b1 does not equal b2. |
 |
If lines are parallel, they share no common points. We saw this when we worked with systems of equations. Parallel systems had no solutions. So if we set these two equations equal, and try to solve for x, there must be no solution. Let's see what happens:
m1x + b1 = m2x + b2
m1x - m2x = b2 - b1
x(m1 - m2) = b2 - b1
Since we are given that m1 = m2 , we know m1 - m2 = 0.
x(0) = b2 - b1
b2 = b1
But, if b2 = b1, the two lines coincide (are the same line).
This is a contradiction to the given that the lines are two distinct lines.
So there is no solution to this system of equations.
The lines p and q must be parallel. |

NOTE: The re-posting of materials (in part or whole) from this site to the Internet
is copyright violation
and is not considered "fair use" for educators. Please read the "Terms of Use". |
|










