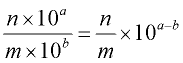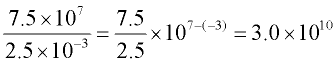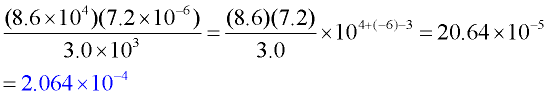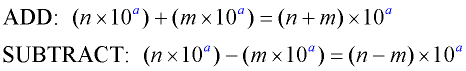Scientific notation is a shorthand method of expressing very large or very small numbers based on powers of the base number 10. Calculators, scientists and engineers find this base 10 method of expressing values to be very helpful, as it allows for very precise calculations.

Scientific notation is a form of exponential notation (using exponents).

Consider the speed of light, 300000000 m/sec. When writing this value, it is very easy to accidentally "lose" one, or more, of the zeros. It is much faster and easier to write:
        3.0 × 108 m/sec   or   3.0 E+8 m/sec.
The picture at the right shows the Hubble Space Telescope as seen from the departing Space Shuttle Atlantis during a servicing mission.
Photo courtesy of NASA.		 hubble

While there are many ways to write values to a power of 10, there is only one format that is accepted as proper "scientific notation".

bullet Proper Scientific Notation:
To convert to scientific notation:
• The value is rewritten to contain a decimal point with ONE non-zero digit to the left of the decimal point.
• This result is then multiplied by a power of 10, where the exponent represents the number of moves necessary to return the value to its original state.
• If the decimal must move to the right, the exponent is positive. If the decimal must move to the left, the exponent is negative.
scipic
Note: Since we are dealing with | a |, the a could be either positive (3×105) or negative (-3×105).
Example:
5.3 x 1024 is proper scientific notation.
74.2 x 10-15 is not proper scientific notation because there are 2 digits preceding the decimal point.

bullet Converting to Scientific Notation:
1. Given: 52,400,000. Convert to scientific notation.
Start by rewriting the number so only one digit is to the left of the decimal point, which will be 5.24. Now, for that value to be equivalent to 52,400,000 the decimal must move 7 places to the right, telling us the needed exponent is 7.
ANSWER:   5.24 x 107
2. Given: 0.000012. Convert to scientific notation.
Start with 1.2. Now, the decimal point needs to move 5 places to the left to get 0.000012, so the exponent is -5.
ANSWER:   1.2 x 10-5
3. Express the speed of light (300,000,000 m/sec) in scientific notation.
Start with 3.0. Now the decimal needs to move 8 places to the right to get 300,000,000, so the exponent is 8.
ANSWER:   3.0 x 108 m/sec

 

bullet Converting from Scientific Notation:
We will be referring to values written as numerals (regular numbers) as "standard notation".
1. Given: 3.805 x 105. Convert to standard notation.
 This number is in scientific notation. The positive exponent indicates that a movement of the decimal point 5 places to the right will show the value in standard (regular number) form.
ANSWER:   380,500
2. Given: 6.5 x 10-6. Convert to standard notation.
 This number is in scientific notation. The negative exponent indicates that a movement of the decimal point 6 places to the left will show the standard form.
ANSWER:   0.0000065
3. Express the half-life of Plutonium (8.0 x 107years) in standard notation.
 This number is in scientific notation. The positive exponent indicates that a movement of the decimal point 7 places to the right will show the standard form.
ANSWER:  80,000,000 yrs.

dashdivider

One of the advantages of scientific notation is its ease of use when performing computations.
Watch the laws of exponents at work!

bullet Multiplying and Dividing Scientific Notation:
To Multiply: scimath1
Multiply the numbers out front and add the exponents.

expin1
scimath2
Notice that the first multiplication did not give the answer in proper scientific notation.


To Divide: scimath3
Divide the numbers out front and subtract the exponents.

expin2
scimath4



expin3
scimath5a
Remember to put the final answer into proper scientific notation.

beware1
REAL WORLD ACCURACY:  The multiplication (or division) of values expressed in scientific notation may result in answers with "more decimal accuracy" than the original values. In real world situations, the multiplication (or division) of values cannot result in answers with a higher level of accuracy than the original values. In fact, the answer cannot be stated to any higher level of accuracy than the number of digits in the least accurate number.

For example, in Example 3 above, the answer is 2.064 x 10-4. Had this been a real-world problem, the accuracy of the answer would not exceed the least accurate listings in the problem of one decimal place. The real-world answer would be 2.1 x 10-4

dashdivider

bullet Adding and Subtracting Scientific Notation:
To ADD or SUBTRACT two numbers in scientific notation, the exponents on the power of 10 must be the same. You may need to "adjust" the numbers, moving them out of proper scientific notation, so the exponents are alike.

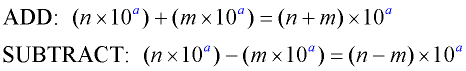
scimath6

expin4
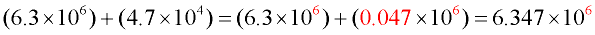
scimath7
The decimal point in the second number was moved two places to the left so that the base of 10 could be raised to a power of 6.


dashdivider

bullet Scientific Notation on the Calculator:
Check to see if your calculator offers a SCI mode (for answers to be expressed in scientific notation). Also, remember that calculators can accept the ^ symbol as an indication of exponent. For example, 53 can be entered as 5 ^ 3. The * signifies x (multiplication).
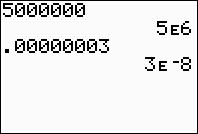calc1
In SCI mode, answers are
displayed in the calculator's
scientific notation format.
5E6 = 5 x 106
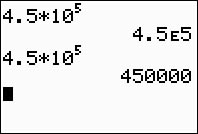calc2
First entry: SCI mode
Second entry: NORMAL mode
ti84c
For help with scientific notation on your calculator,
click here.

divider

NOTE: The re-posting of materials (in part or whole) from this site to the Internet is copyright violation
 and is not considered "fair use" for educators. Please read the "Terms of Use".