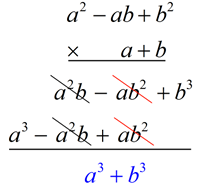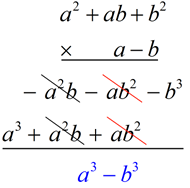|
In Algebra 1, you worked with factoring the differenceof two perfect squares.
a2 - b2 = (a - b)(a + b)
 The sum of two perfect squares, a2 + b2, does not factor under Real numbers. The sum of two perfect squares, a2 + b2, does not factor under Real numbers.
In Algebra 2, we will extend our factoring skills
to
factoring both the difference and the sum of two perfect cubes.
Factor the Sum of Perfect Cubes: |
a3 + b3 = (a + b)(a2 - ab + b2)
|
Factor the Difference of Perfect Cubes: |
a3 - b3 = (a - b)(a2 + ab + b2)
|
 |
When trying to remember these patterns, remember that the first binomial term in the factored form in each pattern keeps the same sign as the sign between the perfect cubes. The sign separating the first and second terms of the trinomial is opposite the sign between the perfect cubes, and the last sign in the trinomial is always positive.
(Note that each formula has only one negative sign in the solution.)
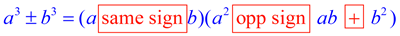
|
If you remember that the first part of the factoring (the binomial part) is the sum (or difference) of a and b,
you can always find the rest of the factored answer by using long division.
One more idea for remembering these patterns:
If you remember one of the patterns,
you can obtain the other pattern by substituting "-b" in place of "b" in your known pattern.
For example, if you know:
a3 + b3 = (a + b)(a2 - ab + b2)
Replace b with -b: a3 + (-b)3 = (a + (-b))(a2 - a(-b) + (-b)2)
and you have: a3 - b3 = (a - b)(a2 + ab + b2)
Let's see that these formulas are actually true.
When working with perfect cubes look for:
perfect cube numerical values: 8, 27, 64, 125,
216, 343, 512, ...
powers for perfect cube algebraic values:  |
|
Factor: 8x3 - 27 |
Is this expression the difference of perfect cubes?
Look for perfect cubes and appropriate powers.
8x3 and 27 are perfect cubes: 8x3 = (2x)3 and 27 = 33
So, yes, this is the difference of perfect cubes.
|
(2x)3 - 33
a = 2x, b = 3 |
Use formula: a3 - b3 = (a - b)(a2 + ab + b2).
Be sure to use parentheses to avoid problems. |
(2x - 3)((2x)2 + (2x)(3) + 32) |
Remove the inner parentheses on the trinomial expression. This is your factored answer. |
(2x - 3)(4x2 + 6x + 9) |

|
Factor: 64x9 + 125 |
64x9 = (4x3)3 and
125 = 53
This expression is the sum of perfect cubes. |
(4x3)3 + 53 |
a = 4x3 and b = 5
formula:
a3 + b3 = (a + b)(a2 - ab + b2) |
(4x3 + 5)((4x3)2 - (4x3)(5)+ 52) |
Simplify the trinomial to get the factored form. |
(4x3 + 5)(16x6- 20x3+ 25)
|
|
Factor: 56m3 - 7n3 |
At first glance, this may not look like the difference of perfect cubes. BUT, if we factor out the common factor of 7, we will find a hidden difference of perfect cubes. |
7(8m3 - n3) |
a = 2m and b = n
Use formula: a3 - b3 = (a - b)(a2 + ab + b2). |
7(2m - n)((4m)2 + 2mn + n2) |
Solution: |
7(2m - n)(4m2 + 2mn + n2)
|

Let's take a look at cubes of binomials.
Cubing a binomial: |
(x + a)3 = x3 + 3ax2 +3a2x + a3
|
You can obtain this next pattern by substituting "-a" for "a": |
(x - a)3 = x3 - 3ax2 +3a2x - a3
|
When you work with the Binomial Theorem, you will get a better understanding
of the pattern of the coefficients in these formulas.
Remember: you can always find these patterns by simply multiplying
(x + a)3 = (x + a)(x + a)( x + a)
(x - a)3 = (x - a)(x - a)( x - a)

NOTE: The re-posting of materials (in part or whole) from this site to the Internet
is copyright violation
and is not considered "fair use" for educators. Please read the "Terms of Use". |
|
|